1738: Hydrodynamica and Bernoulli’s Principle

Author David Poole on 4 December 2017 David Poole's blog

In fluid dynamics, Bernoulli’s principle, a particular example of the conservation of energy, states that an increase in the speed of a fluid occurs simultaneously with a decrease in pressure or a decrease in the fluid’s potential energy. The principle is named after Basel based mathematician Daniel Bernoulli who published it in his book Hydrodynamica in 1738. Bernoulli is regarded as the founding father of fluid dynamics. A consequence of his principle is that if the velocity increases then the pressure falls. This is exploited by the wing of an aircraft, which is designed to create an area of fast flowing air above its surface. The pressure of this area is lower and so the wing is pulled upwards.

Bernoulli built much of his work off that of Newton.  In 1738, he published Hydrodynamica (link to the original book), his study of how fluids behave when they are in motion. Even if you cannot read Latin (like myself), it is awe inspiring to browse through this historical book.

Although air is a gaseous substance, it is also considered a fluid because it flows and can take on different shapes. Bernoulli asserted in Hydrodynamica that as a fluid moves faster, it produces less pressure, and conversely, slower moving fluids produce greater pressure. In the tenth chapter, Bernoulli discussed the first model of the kinetic theory of gases. Assuming that heat increases the velocity of the gas particles, he demonstrated that the pressure of air is proportional to the kinetic energy of gas particles, thus making the temperature of gas proportional to this kinetic energy as well.

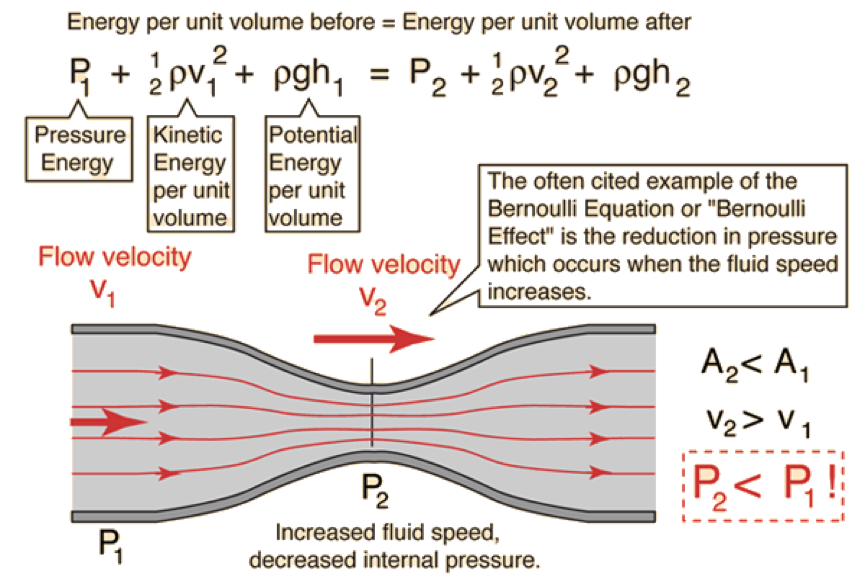
The Bernoulli Equation is equivalent to Newton’s conservation of energy principle applied to flowing fluids. The “Bernoulli effect” is the lowering of fluid pressure in regions where the flow velocity is increased. This lowering of pressure in a constriction of a flow path may seem counter-intuitive, but not when you consider pressure to be energy density. In the high velocity flow through the constriction, kinetic energy must increase at the expense of pressure energy. The Bernoulli Equation applied to a constriction in a tube is illustrated below. Here, P is pressure, ρ is the density of the fluid, v its velocity, A the cross-sectional area of the tube and h the height of the fluid at a given point.

Bernoulli discovers how to measure blood pressure

Daniel Bernoulli collaborated with his father’s student Leonhard Euler, who also came from Basel. Together Bernoulli and Euler tried to find out more about the relationship between the speed at which blood flows and its pressure. To investigate this, Bernoulli experimented by puncturing the wall of a pipe with a small open-ended straw and noted that the height to which the fluid rose up the straw was related to fluid’s pressure in the pipe.

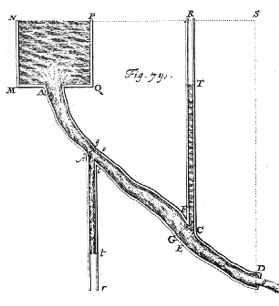
Soon physicians all over Europe were measuring patients’ blood pressure by inserting pointed glass tubes directly into their arteries. It was not until about 170 years later, in 1896 that an Italian doctor discovered a less painful method which is still in use today. However, Bernoulli’s method of measuring pressure is still used today in modern aircraft to measure the speed of the passing air i.e. its air speed.

About Daniel Bernoulli (1700–1782)

Daniel Bernoulli was one of the Basel based family of famous mathematicians and is the founding father of fluid dynamics. Other famous Bernoulli family members include Johann who was one of the earliest discoverers of calculus and Jacob Bernoulli who pioneered the theory of probability.

References and further reading

1. Wikipedia: https://en.wikipedia.org/wiki/Conservation_of_energy
2. Wikipedia:https://en.wikipedia.org/wiki/Daniel_Bernoulli
3. ETH Bibliothek: https://www.e-rara.ch/doi/10.3931/e-rara-3911
4. Wikipedia: https://en.wikipedia.org/wiki/Kinetic_theory_of_gases
5. Plus Magazine: https://plus.maths.org/content/daniel-bernoulli-and-making-fluid-equation
6. Wikipedia:https://en.wikipedia.org/wiki/Leonhard_Euler